This contest has ended. Thanks to everyone who helped track down these questions! Now onto round three.
The 2014-15 USAMTS Round Two problem set is out, and it appears that there are numerous questions from it lurking on the main site: I have found about ten so far today, in addition to a few that were flagged by users.
This is a request for all users to be on the lookout for these problems, and to flag them for moderator attention when you see them so that we can lock them as per our policy on ongoing contest problems. The current problem set sheet can be found here, and I transcribe the problems below:
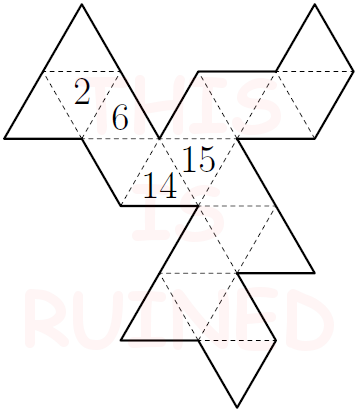
The net of $20$ triangles shown to the right can be folded to form a regular icosahedron. Inside each of the triangular faces, write a number from $1$ to $20$ with each number used exactly once. Any pair of numbers that are consecutive must be written on faces sharing an edge in the folded icosahedron, and additionally, 1 and 20 must also be on faces sharing an edge. Some numbers have been given to you.
You do not need to prove that your answer is the only one possible; you merely need to find an answer that satisfies the constraints above. (Note: In any other USAMTS problem, you need to provide a full proof. Only in this problem is an answer without justification acceptable.)
Let $a,b,c,x,y$ be positive real numbers such that $$ax + by \leq bx + cy \leq cx + ay.$$ Prove that $b \leq c$.
Let $\mathcal{P}$ be a square pyramid whose base consists of the four vertices $(0, 0, 0)$, $(3, 0, 0)$, $(3, 3, 0)$, and $(0, 3, 0)$, and whose apex is the point $(1, 1, 3)$. Let $\mathcal{Q}$ be a square pyramid whose base is the same as the base of $\mathcal{P}$, and whose apex is the point $(2, 2, 3)$. Find the volume of the intersection of the interiors of $\mathcal{P}$ and $\mathcal{Q}$.
A point $P$ in the interior of a convex polyhedron in Euclidean space is called a pivot point of the polyhedron if every line through $P$ contains exactly $0$ or $2$ vertices of the polyhedron. Determine, with proof, the maximum number of pivot points that a polyhedron can contain.
Find the smallest positive integer $n$ that satisfies the following: We can color each positive integer with one of $n$ colors such that the equation $$w + 6x = 2y + 3z$$ has no solutions in positive integers with all of $w, x, y, z$ the same color. (Note that $w, x, y, z$ need not be distinct: for example, $5$ and $7$ must be different colors because $(w, x, y, z) = (5, 5, 7, 7)$ is a solution to the equation.)