103
$\begingroup$

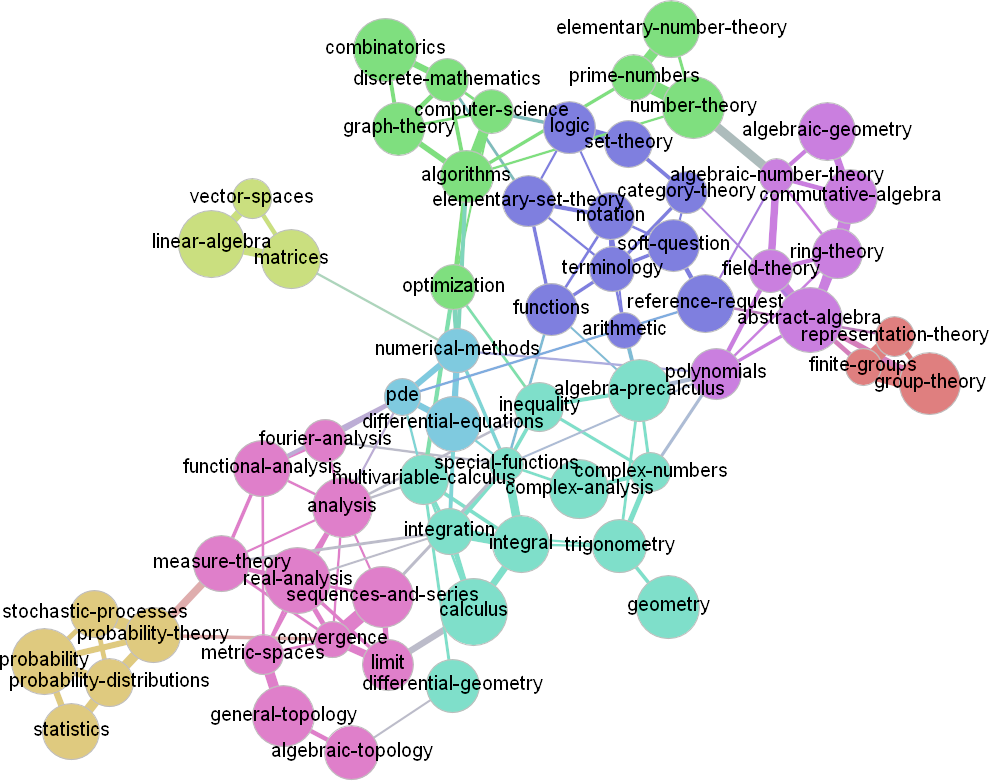
I've just made a map of tags for Math.SE. (And to some degree - a greatly simplified map of mathematics.)

In short: tag size is related to tag popularity (caveat: see below) and edges are related to tag co-occurrences in questions (or more precisely: the observed/expected ratio, see About joint probability divided by the product of the probabilities). Colors are to distinguish graph communities, as detected by this algorithm.

For me it looks as a "snapshot" of topics and scope of this SE site. So if you want to use it in any way to promote Math.SE - feel free!

Also, if you have comments how to improve its usefulness or niceness to our community, I would appreciate them (but bear in mind that I have no color esthetics).

Tag Map for Math.SE - Gephi ARF

Caveat: I plotted all of 64 most popular tags that have at least one link, that is, 63 tags. As I see now, (the most popular tag) went out. Lousily speaking, it means that this tag doesn't co-occur with other tags "much more than on random". Do you think that I should attach it as a separate node? (Alternatively, I can lower the threshold, but then (almost) everything is going to be linked with almost everything, hardly making in any clearer.)

Edit:

I added one more visualization, this time a bit less dense. Based on Gephi - OpenOrd:

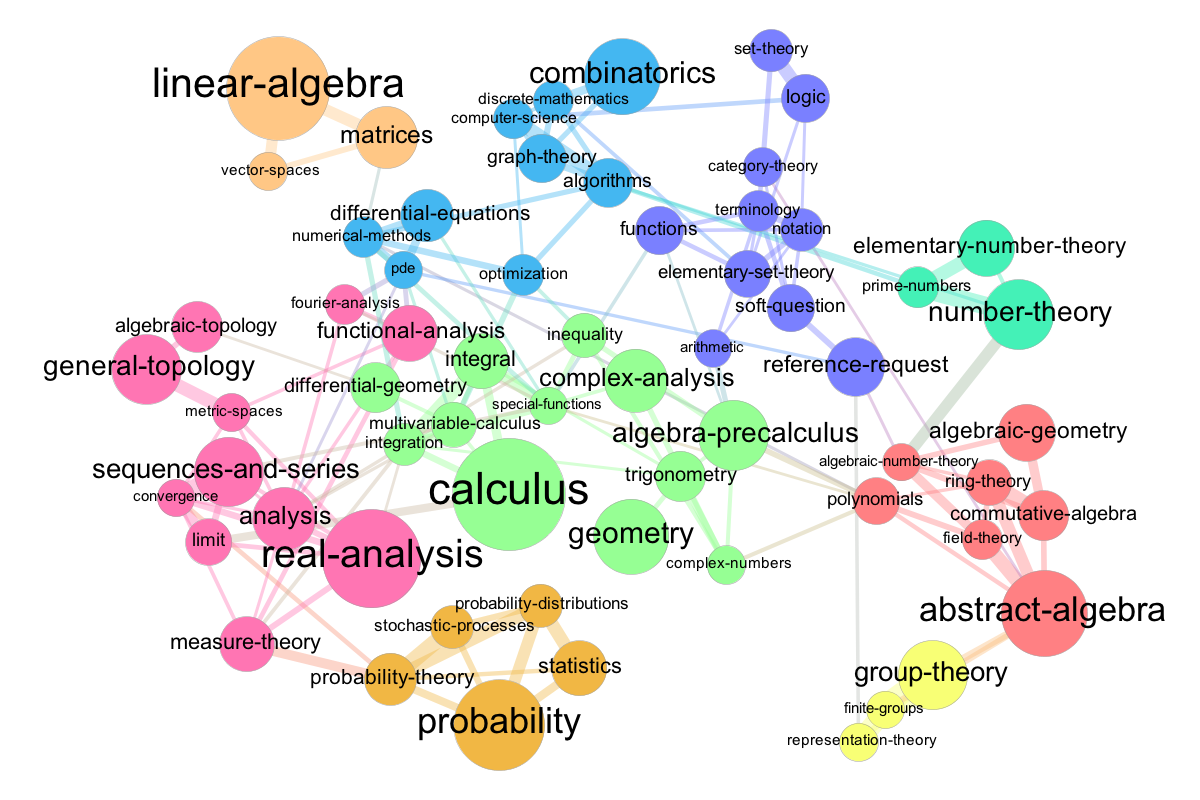
Tag Map for Math.SE - Gephi OpenOrd

Links:

$\endgroup$
13
  • 6
    $\begingroup$ I like this a lot - it's very interesting. But I notice that it can't quite be accurate because there is not a homework bubble. But then again, perhaps it wouldn't fit. $\endgroup$
    – davidlowryduda Mod
    Commented Nov 1, 2012 at 17:33
  • 2
    $\begingroup$ @mixedmath I plotted all of 64 most popular tags that have at least one link, that is, 63 tags. As I see now, homework (the most popular tag) went out. Lousily speaking, it means that this tag doesn't co-occur with other tags "much more than on random". Do you think that I should attach it as a separate node? (Alternatively, I can lower the threshold, but then (almost) everything is going to be linked with almost everything, hardly making in any clearer.) $\endgroup$
    – Piotr Migdal
    Commented Nov 1, 2012 at 17:42
  • 2
    $\begingroup$ No, I was saying it sort of as a comment on how much homework there is - I really like the graph as it is. $\endgroup$
    – davidlowryduda Mod
    Commented Nov 1, 2012 at 17:51
  • $\begingroup$ So, "numerical methods" are the center of Mathematics? It's a little odd to see "stochstic processes" surrounded by Number Theory topics, and to see "graph theory" blocking the view of "algebraic number theory" from "number theory", but I guess these things can't be helped in two dimensions. Nice diagram. $\endgroup$
    – Gerry Myerson
    Commented Nov 1, 2012 at 22:53
  • $\begingroup$ @Gerry, I think it's also an artifact of the fact that the nodes are being "pushed together" to form a neat circle instead of having enough space to keep away from unrelated nodes. After all, "stochastic processes" has no edges connecting it to any number theory tags. Piotr, can you lift the circle packing condition, or try a different layout algorithm? It's a really nice visualization, and the clustering shown in the connectivity and the colours does make a lot of sense. $\endgroup$
    – user856
    Commented Nov 1, 2012 at 23:04
  • $\begingroup$ @RahulNarain The compression + using colors was intentional. If it is too sparse, then names are smaller (because lower fraction of space is used). Also, it's only 2D embedding (I would love to use 63 dimensions ;)). However, how about this (Force Atlas-based) or (maybe even better) that (OpenOrd-based)? $\endgroup$
    – Piotr Migdal
    Commented Nov 1, 2012 at 23:34
  • $\begingroup$ I think I like those two more than the original. $\endgroup$
    – Gerry Myerson
    Commented Nov 2, 2012 at 5:30
  • $\begingroup$ I like the OpenOrd based one best. But even there some of the spacings are non-intuitive to me. Of course I realise that projections from 63 dimensional space to 2D necessarily lose information. Great job there! $\endgroup$
    – Willie Wong Mod
    Commented Nov 2, 2012 at 9:48
  • $\begingroup$ What would happen if you manually remove/ignore all of the meta-tags? (notation, reference-request, terminology, soft-question) $\endgroup$
    – user642796
    Commented Nov 2, 2012 at 13:38
  • $\begingroup$ @ArthurFischer More-or-less the same plot, but without that tags. For sake of this visualization a tried to focus rather on Math.SE than mathematics itself (anyway, connections are based only on questions here, which may be different from other measures, e.g. historical influence, mathematicians working in both disciplines, usage of theorems from other, books on join topics, etc). And I tried avoid manual manipulation as much as possible (except for some cosmetic position adjustments). But yes, it may be worth trying. $\endgroup$
    – Piotr Migdal
    Commented Nov 2, 2012 at 14:59
  • $\begingroup$ It might also be interesting to see separate graphs of those clusters, with just those extra topics that some topic in the cluster direcftly links to. $\endgroup$
    – Tobias Kildetoft
    Commented Nov 2, 2012 at 20:45
  • $\begingroup$ Is there an analogue of this map for Mathoverflow? $\endgroup$
    – Dominik
    Commented Jun 30, 2013 at 21:18
  • $\begingroup$ @Dominik Not yet. I was thinking about it. Now, as they have SE2.0 API it will be easy for me (or anyone using the script) to generate plots from MathOverflow. However, I am planning to make something better (doing plots of the same time gets boring after some time) providing more interaction and added value. $\endgroup$
    – Piotr Migdal
    Commented Jul 1, 2013 at 21:11

1 Answer 1

Reset to default
37
$\begingroup$

I played with the layout settings and made this version:

enter image description here

I feel like with this layout you can more clearly see the shape of the connectivity between tags. For example, the arrangement seems to be stretched out along a discrete/continuous spectrum. But on the discrete side, there isn't a lot of overlap between the algebraists and the computer-sciencey types.

$\endgroup$
5
  • 10
    $\begingroup$ Nice. Odd, though, to see linear algebra so far from abstract algebra. $\endgroup$
    – Gerry Myerson
    Commented Nov 2, 2012 at 11:28
  • 3
    $\begingroup$ This one is nice (and for some reason reminds me of World of Goo :)). And I'm happy to see you playing with it. $\endgroup$
    – Piotr Migdal
    Commented Nov 2, 2012 at 12:25
  • 1
    $\begingroup$ So odd that the Linear algebra cluster is all out on it's own. A priori I would have thought it'd be the most connected. $\endgroup$
    – Nick Alger
    Commented Nov 3, 2012 at 8:47
  • $\begingroup$ @NickAlger It's matter of metrics. Here ($P(A\cap B)/[P(A)P(B)]$ the most correlated tags. If a tags is seen with a lot of things, but is not very specific, then there are little edges (compare: homework). If you want $\max(P(A|B),P(B|A))$ then some nodes are connected with almost everything, and there is a lot of nodes not connected to anything (actually, it was my initial, failed approach for StackOverflow). However, I have idea to cover it, even detecting tag hierarchy, so stay tuned. $\endgroup$
    – Piotr Migdal
    Commented Nov 3, 2012 at 11:41
  • $\begingroup$ @NickAlger And BTW, see linear-algebra -> related tags; it is one of the most common tags, but does not come that often with others, except for matrices and vector-spaces. abstract-algebra is the next, but was it was below the cut-off. $\endgroup$
    – Piotr Migdal
    Commented Nov 6, 2012 at 16:05

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .